CASIMIR EFFECT
PEOPLE
Romain Guérout
Astrid Lambrecht
Serge Reynaud
In its modern quantum conception, space is filled by vacuum field fluctuations which have observable consequences such as radiative corrections or spontaneous emission. These vacuum fluctuations have also mechanical effects in macrophysics, in particular the Casimir forces. Measurements of Casimir force between gold mirrors now reach a good experimental precision, but the comparison with theoretical predictions still raises difficulties.
This comparison has interesting connections with open questions in fundamental physics. The static Casimir effect is related with the puzzles of gravitational physics through the problem of vacuum energy while the dynamic Casimir effect shows the limit of the principle of relativity of motion associated with the classical theory of relativity. Casimir physics also plays an important role in the tests of gravity at short ranges.
The notes of the lectures at the 101th Les Houches Summer School “Quantum Optics and Nanophotonics” in August 2013 present the main features of the theory of Casimir forces for 1-dimensional model systems and for mirrors in 3-dimensional space.
- Casimir forces, S. Reynaud, A. Lambrecht, in Quantum Optics and Nanophotonics, Fabre C., Sandoghdar V., Treps N. and Cugliandolo L. eds (Oxford University Press, 2017) pp. 407-455 [arXiv version]
Casimir effect in the scattering formalism
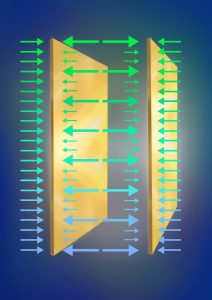
Schematic representation of the Casimir force as the result of vacuum radiation pressure summed over all field modes.
The coupling of arbitrary objects with electromagnetic fluctuations is described by scattering matrices containing reflection and transmission amplitudes which depend on frequency, wave-vector and polarization. The Casimir energy is the change of vacuum energy while the Casimir force is the sum over all field modes of the radiation pressure of vacuum fluctuations.
This scattering method can be applied to the calculation of forces between arbitrary mirrors (plane, spherical, nanostructured, rough) as well as atoms or molecules near a surface or other atoms, molecules or nanoobjects. The scattering approach has been developed and applied to the Casimir effect with an increasing degree of generality in the following papers:
- Casimir force between partially transmitting mirrors, M.-T. Jaekel, S. Reynaud, Journal de Physique I 1, 1395 (1991)
- Casimir force between metallic mirrors, A. Lambrecht, S. Reynaud, Eur. Phys. J. D8, 309 (2000)
- The Casimir force and the quantum theory of lossy optical cavities, C. Genet, A. Lambrecht, S. Reynaud, Phys. Rev. A 67, 043811 (2003)
- The Casimir effect within scattering theory, A. Lambrecht, P.A. Maia-Neto, S. Reynaud, New J. of Phys. 8, 243 (2006)
- Derivation of the Lifshitz-Matsubara sum formula for the Casimir pressure between metallic plane mirrors, R. Guérout, A. Lambrecht, K.A. Milton, S. Reynaud, Phys. Rev. E. 90, 042125 (2014)
- Accounting for dissipation in the scattering approach to the Casimir energy, R. Guérout, G.-L. Ingold, A. Lambrecht, S. Reynaud, Symmetry 10, 37 (2018)
Material properties and Casimir effect
Common configuration for the calculation of Casimir force : plane-plane geometry between two gold-covered mirrors.
Mirrors used in most experiments are metallic and the Casimir force is affected significantly by their finite conductivity. Large distances correspond mainly to low frequencies for which the metallic mirrors are nearly perfectly reflecting, so that the Casimir force approaches the force calculated for ideal mirrors. This approach however shows subtle properties for dissipative media at non zero temperature.
- Quantum dissipative Brownian motion and the Casimir effect, G-L. Ingold, A. Lambrecht, S. Reynaud, Phys. Rev. E. 80, 041113 (2009)
- Derivation of the Lifshitz-Matsubara sum formula for the Casimir pressure between metallic plane mirrors, R. Guérout, A. Lambrecht, K.A. Milton, S. Reynaud, Phys. Rev. E. 90, 042125 (2014)
For experiments performed between metallic plane mirrors, the commonly used expression is valid for the lossy model of optical response, but not for the lossless plasma model. In fact, the modes associated with the Foucault currents play a crucial role in the limit of vanishing losses.
- Lifshitz-Matsubara sum formula for the Casimir pressure between magnetic metallic mirrors, R. Guérout, A. Lambrecht, K.A. Milton, S. Reynaud, Phys. Rev. E. 93, 022108 (2016)
For metallic mirrors at short distances (below one micron) associated with high-frequency modes, the metallic mirrors behave as poor reflectors and the effect of finite conductivity reduces the Casimir force.
- Casimir force between metallic mirrors, A. Lambrecht and S. Reynaud, Eur. Phys. J. D8, 309 (2000)
These theoretical results show the importance of a full comprehension of the influence of the material properties of the mirrors on the Casimir forces.
- Quantitative non contact dynamic Casimir force measurements, G. Jourdan, A. Lambrecht, F. Comin, J. Chevrier, EPL 85, 31001 (2009)
- Influence of slab thickness on the Casimir force, I. Pirozhenko and A. Lambrecht, Phys. Rev.A 77, 013811 (2008)
- Casimir force between a metal and a semimetal, G. Torricelli, I. Pirozhenko, S. Thornton, A. Lambrecht, C. Binns, EPL 93 (2011) 51001
- Measurement of the Casimir effect under ultrahigh vacuum: Calibration method, G. Torricelli, S. Thornton, C. Binns, I. Pirozhenko, and A. Lambrecht J. Vac. Sci. Technol. B 28, C4A30 (2010)
At very short distances, the Casimir force corresponds directly to the instantaneous interaction between the surface plasmons living on the two metallic surfaces. Surface plasmons produce a repulsive contribution to the force for median and large distances, while the global force remains attractive. It can thus be expected that the Casimir forces may be affected by changing the coupling between plasmons and photons through nanostructured surfaces.
![]() Surface plasmon modes and the Casimir energy, F. Intravaia, A. Lambrecht, Phys. Rev. Lett. 94 (2005) 110404
Surface plasmon modes and the Casimir energy, F. Intravaia, A. Lambrecht, Phys. Rev. Lett. 94 (2005) 110404
![]() The Role of Surface Plasmons in The Casimir Effect, F. Intravaia, C. Henkel, A. Lambrecht, Phys. Rev. A 76 (2007) 033820
The Role of Surface Plasmons in The Casimir Effect, F. Intravaia, C. Henkel, A. Lambrecht, Phys. Rev. A 76 (2007) 033820
![]() Repulsive Casimir forces and the role of surface modes, I. Pirozhenko, A. Lambrecht, Phys. Rev; A 80 (2009) 042510
Repulsive Casimir forces and the role of surface modes, I. Pirozhenko, A. Lambrecht, Phys. Rev; A 80 (2009) 042510
Casimir forces in the plane-sphere geometry
Common configuration for measurements of Casimir force : plane-sphere geometry between two gold-covered mirrors.
Most precise experiments measure the force between a plane and a sphere in vacuum or thermal electromagnetic fields. With the scattering formula developed on adapted plane-wave and spherical-wave basis, we have obtained expressions valid for arbitrary values of the sphere radius, inter-plate distance and plasma wavelength. These calculations go beyond the proximity force approximation, which was used for analyzing most experiments.
These calculations of the Casimir force took into account for the first time geometry effects as well as material properties of the plates (gold in most experiments).
- Casimir Interaction between Plane and Spherical Metallic Surfaces, A. Canaguier-Durand, P.A. Maia Neto, I. Cavero-Pelaez, A. Lambrecht, S. Reynaud, Phys. Rev. Lett. 102, 230404 (2009)
- Casimir energy between a plane and a sphere in electromagnetic vacuum, P.A. Maia Neto, A. Lambrecht, S. Reynaud, Phys. Rev. A 78 012115 (2008)
In the case of a non zero temperature, negative interaction entropies were predicted with a contribution of thermal photons to the Casimir force possibly repulsive. The full electromagnetic results depart from previously used approximations significantly more rapidly than expected from existing scalar computations.
- Thermal Casimir Effect in the Plane-Sphere Geometry, A. Canaguier-Durand, P.A. Maia Neto, A. Lambrecht, S. Reynaud, Phys. Rev. Lett. 104, 040403 (2010)
- Thermal Casimir effect for Drude metals in the plane-sphere geometry A. Canaguier-Durand, P.A. Maia Neto, A. Lambrecht, S. Reynaud, Phys. Rev. A 82, 012511 (2010)
In the classical limit attained at high temperatures, the Casimir interaction in the plane-sphere geometry between two Drude metals tends to be independent of the conductivity, which is not the case with material properties modeled by a plasma model. The Drude model has thus a more universal character than the plasma model. Interestingly the development in the vicinity of the local approximation cannot be represented by an analytical series in the aspect ratio characterizing the geometry.
- Classical Casimir interaction in the plane-sphere geometry, A. Canaguier-Durand, G.-L. Ingold, M.-T. Jaekel, A. Lambrecht, P.A. Maia Neto, S. Reynaud, Phys. Rev. A 85, 052501 (2012)
Theory-experiment comparison
The Casimir effect is an important prediction of Quantum Field Theory, which deserves a careful experimental verification. The comparison between experiments and theory is also important as a tool to derive constraints on hypothetical new short-range interactions appearing besides the gravity force in unification models. A review bearing on gravity tests at sub-mm scales can be found in
- Short-range fundamental forces, I. Antoniadis, S. Baessler, M. Buechner, V.V. Fedorov, S. Hoedl, A. Lambrecht, V.V. Nesvizhevsky, G. Pignol, K.V. Protasov, S. Reynaud, Y. Sobolev, C. R. Phys. 12 755-778 (2011)
The comparison between experiments and theory has to account for the differences between the ideal case initially discussed by Casimir and the recent accurate experiments. These experiments use imperfectly reflecting mirrors (not perfect ones), in the plane-sphere geometry (not in the more easily calculated plane-plane geometry), and at room temperature (thermal fluctuations to be taken into account besides vacuum fluctuations). Meanwhile, the surfaces of the mirrors are not perfect as they show roughness (topographic irregularities) and patches (voltage irregularities). We have worked on many of these effects in order to assess the significance of the unexpected discrepancy observed between experiments and theory. Recent measurements are indeed agreeing with the lossless plasma model while excluding the better motivated dissipative Drude model (which describes the finite conductivity of metals such as gold).
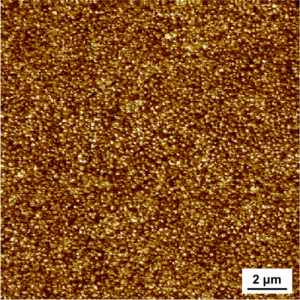
Distribution of the irregular patch potentials measured by KPFM on gold samples similar to those used in Casimir experiments.
In collaboration with colleagues at Los Alamos National Laboratory and UF Rio de Janeiro, we have analyzed the patch contribution to Casimir experiments with a model of quasi-local voltage correlations, derived from well-motivated physical principles. This model could have been used to fit the difference between theory and the experimental data of the IUPUI experiment (at this stage, it was only a fit, not an explanation of the discrepancy).
- Modeling electrostatic patch effects in Casimir force measurements, R. O. Behunin, F. Intravaia, D. A. R. Dalvit, P. A. Maia Neto, S. Reynaud Phys. Rev. A 85, 012504 (2012)
We also performed Kelvin probe force microscopy with two different groups on the Au-coated planar samples used to measure the Casimir interaction at IUPUI. When using the measured voltage distribution to calculate the separation dependence of the patch pressure in the plane-sphere geometry, we found that patches did not explain the magnitude or the separation dependence of the difference between the measured Casimir pressure and the one calculated using a Drude model.
- Kelvin probe force microscopy of metallic surfaces used in Casimir force measurements, RO Behunin, DAR Dalvit, RS Decca, C Genet, IW Jung, A Lambrecht, A Liscio, D Lopez, S Reynaud, G Schnoering, G Voisin and Y Zeng Phys. Rev. A 90, 062115 (2014)
- Electrostatic patch effects in Casimir-force experiments performed in the sphere-plane geometry, R.O. Behunin, Y. Zeng, D. A. R. Dalvit, S. Reynaud Phys. Rev. A 86, 052509 (2012)
Casimir forces and nanophysics
Nano-structured surfaces have gained considerable interest in nanophysics. The effects of thermal and quantum fluctuations, in particular through Casimir-like forces, play an important role at the scale of these systems.
The scattering approach allows one to calculate the Casimir interaction energy between arbitrary objects. This method was first applied to evaluate the force between periodic dielectric gratings within the project ANR Pnano MONACO with the LETI/CEA. In contrast to the case of specular reflection on a plane, scattering on a grating changes the wave-vector and polarization of the field. As the scattering couple different diffraction orders, the reflection and transmission matrices are obtained by solving a system of coupled differential equations the number of which is proportional to the number of diffraction orders that need to be considered.
This method has allowed us to compare with theory the Casimir force measurements performed between a Silicon grating and a Gold sphere in the group of H.B. Chan (Phys. Rev. Lett. 101, 030401, 2008 & Phys. Rev. Lett. 105, 250402, 2010).
- Casimir interaction between dielectric gratings, A. Lambrecht, V.N. Marachevsky, Phys. Rev. Lett. 101, 160403 (2008)
- Casimir Force on a Surface with Shallow Nanoscale Corrugations : Geometry and Finite Conductivity Effects, Y. Bao, R. Guérout, J. Lussange, A. Lambrecht, R. A. Cirelli, F. Klemens, W. M. Mansfield, C. S. Pai, H. B. Chan, Phys. Rev. Lett. 105, 250402 (2010)
- Thermal Casimir force between nanostructured surfaces, R. Guérout, J. Lussange, H. B. Chan, A. Lambrecht, S. Reynaud, Phys. Rev. A 87, 052514 (2013)
Above nano-structured surfaces, the translational invariance of vacuum in transverse direction is broken and a lateral Casimir force arises. It is often calculated through the simple Proximity Force Approximation (PFA) where force contributions at different distances are added as if they were independent from each other. This approximation leads to wrong results for corrugated surfaces with corrugation wavelength smaller than the other relevant length scales.
- Lateral Casimir force beyond the proximity force approximation, R.B. Rodrigues, P.A. Maia Neto, A. Lambrecht, S. Reynaud, Phys. Rev. Lett. 96, 100402 (2006)
- Lateral Casimir force beyond the proximity force approximation: A nontrivial interplay between geometry and quantum vacuum, R. B. Rodrigues, P. A. Maia Neto, A. Lambrecht, S. Reynaud, Phys. Rev. A 75, 062108 (2007)
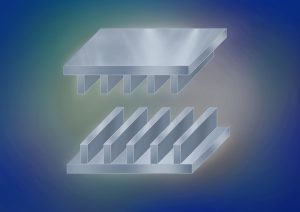
Schematic representation of a configuration with two nano-corrugated plates facing each other. When the corrugations are not aligned, a Casimir torque arises.
When two corrugated metallic plates are placed in vacuum, their interaction with electromagnetic vacuum fields produce a torque which tends to align the corrugations. This Casimir torque could be measured with torsion pendulum techniques for separation distances as large as 1$\mu$m. It would thus allow one to probe the nontrivial geometry dependence of the Casimir energy in a configuration which can be evaluated theoretically with accuracy. In the optimal experimental configuration, the commonly used proximity force approximation turns out to overestimate the torque by a factor 2 or larger.
- Casimir torque between corrugated metallic plates, R. B. Rodrigues, P. A. Maia Neto, A. Lambrecht, S. Reynaud, J. Phys. A 41 164019 (2008)
- Vacuum-induced torque between corrugated metallic plates, R.B. Rodrigues, P.A. Maia Neto, A. Lambrecht, S. Reynaud, Europhys. Lett. 76, 822 (2006)
- Casimir torque between nanostructured plates, R. Guérout, C. Genet, A. Lambrecht, S. Reynaud, EPL 111, 44001 (2015)
Quantum thermodynamics and the Casimir effect
The calculation of Casimir effect at non-zero temperature involves the effect of thermal fluctuations besides that of quantum vacuum fluctuations. In the plane-plane geometry, dissipative mirrors described by the Drude model give rise to a negative interaction entropy which does not appear with the non-dissipative plasma model.
- Quantum dissipative Brownian motion and the Casimir effect, G.-L. Ingold, A. Lambrecht, and S. Reynaud, Phys. Rev. E 80, 041113 (2009)

Ratio of Casimir force at room temperature to the zero temperature force, computed between perfectly reflecting sphere and plane. Solid lines from bottom to top correspond to increasing values of sphere radii. The upper dashed curve represents the PFA expression while the lower dotted curve is the analytical asymptotic expression for L≫R.
In the sphere-plane geometry the Casimir interaction may also produce negative entropy even for perfectly reflective mirrors. This is seen on the figure as the fact that the force can be smaller at room temperature than at zero temperature. The proximity force approximation (dashed line on the figure), based on a calculation of the force in the plane-plane geometry, does not match this effect which is intrinsically linked to geometry.
- Thermal Casimir Effect in the Plane-Sphere Geometry, A. Canaguier-Durand, P.A. Maia Neto, A. Lambrecht, and S. Reynaud, Phys. Rev. Lett. 104, 040403 (2010)
- Thermal Casimir effect for Drude metals in the plane-sphere geometry A. Canaguier-Durand, P.A. Maia Neto, A. Lambrecht, and S. Reynaud, Phys. Rev. A 82, 012511 (2010)
The latter effect is most pronounced in the dipole approximation, which is reliable when the size of the sphere is small compared to the separation between the sphere and the plate. Negative entropies can occur in many different situations: between two electrically and magnetically polarizable nano-particles or atoms, which need not be isotropic, and between such a small object and a conducting plate; between two perfectly conducting spheres, between two electrically polarizable nano-particles if there is sufficient anisotropy, between a perfectly conducting sphere and a Drude sphere, and between a sufficiently anisotropic electrically polarizable nano-particle and a transverse magnetic conducting plate.
- Negative Casimir entropies in nanoparticle interactions, K.A. Milton, R. Guérout, G.-L. Ingold, A. Lambrecht, S. Reynaud, J. Phys. Condensed Mat. 27, 214003 (2015)
- Geometric origin of negative Casimir entropies: A scattering-channel analysis, G.-L. Ingold, S. Umrath, M. Hartmann, R. Guérout, A. Lambrecht, S. Reynaud, K.A. Milton, Phys. Rev. E 91, 033203 (2015)