Non-local quantum states
Recent Publications
♦ Alternative experimental ways to access entropy production
Z. Tan, P. A. Camati, G. Cœuret Cauquil, A. Auffèves, and I. Dotsenko
Phys. Rev. Research 3, 043076 (2021)
♦ Autonomous Maxwell’s demon in a cavity QED system
B.-L. Najera-Santos, P. A. Camati, V. Métillon, M. Brune, J.-M. Raimond, A. Auffèves, and I. Dotsenko
Phys. Rev. Research 2, 032025(R) (2020)
♦ Benchmarking Maximum-Likelihood State Estimation with an Entangled Two-Cavity State
V. Métillon, S. Gerlich, M. Brune, J.-M. Raimond, P. Rouchon, and I. Dotsenko
Phys. Rev. Lett. 123, 060404 (2019)
♦ Measurement of a microwave field amplitude beyond the standard quantum limit
M. Penasa, S. Gerlich, T. Rybarczyk, V. Métillon, M. Brune, J. M. Raimond, S. Haroche, L. Davidovich and I. Dotsenko
Phys. Rev. A 94, 022313 (2016)
♦ Forward-Backward analysis of the photon-number evolution in a cavity
T. Rybarczyk, B. Peaudecerf, M. Penasa, S. Gerlich, B. Julsgaard, K. Moelmer, S. Gleyzes, M. Brune, J.M. Raimond, S. Haroche and I. Dotsenko
Phys. Rev. A 91, 062116 (2015)
♦ Adaptive Quantum Nondemolition Measurement of a Photon Number
B. Peaudecerf, T. Rybarczyk, S. Gerlich, S. Gleyzes, J. M. Raimond, S. Haroche, I. Dotsenko, and M. Brune
Phys. Rev. Lett. 112, 080401 (2014)
♦ Quantum feedback experiments stabilizing Fock states of light in a cavity
B. Peaudecerf, C. Sayrin, X. Zhou, T. Rybarczyk, S. Gleyzes, I. Dotsenko, J. M. Raimond, M. Brune, and S. Haroche
Phys. Rev. A 87, 042320 (2013)
PEOPLE Permanents: Igor Dotsenko, Michel Brune, Jean-Michel Raimond, Serge Haroche
Postdocs: Stefan Gerlich, Zheng Tan
PhDs: Guillaume Cœuret-Cauquil, Mariane Penasa, Valentin Métillon
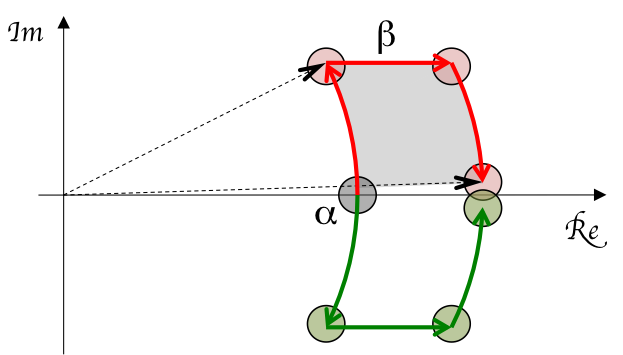
Quantum non-demolition measurement and non-local physics with atoms and cavities
A superconducting high-quality cavity allows us to store a microwave field of several photons for a fraction of second without losing them. Coupling this field to a stream of atoms, excited to high-lying Rydberg states and crossing the cavity one by one, makes it possible to realise many thought experiments proposed by the founders of quantum mechanics to illustrate its highly non-intuitive consequences. Our experimental setup implements a very simple quantum system and allows us to perform fundamental experiments. They contribute to a better understanding of the quantum properties of microscopic objects and of their absence in the classical macroscopic world.
With long cavity damping times, we can store photons long enough to count them without destroying, thus realising a quantum non-demolition measurement of their number. So far, a series of experiments with one cavity based on non-demolition photon counting technique has been realised. Among them, full reconstruction of quantum states of light, quantum feedback stabilising photon-number states, adaptive quantum measurements, analysis of past quantum states, etc.
In the following, we extend the study of quantum phenomena to the non-local physics, present in the micro-world and absent in the macro-world. We have upgraded our experimental setup to two cavities and extend the developed methods and properties of local quantum measurements to their non-local counterparts. This is realised by letting the atoms to interact successively with both cavity fields, thus entangling them. After this operation, the two fields cannot be treated separately and should be considered as one non-local quantum-mechanical object. Some exotic states, e.g. with all photons simultaneously only in the first cavity and only in the second one, can thus be prepared and studied. The ultimate aim of the project is to study quantum-to-classical transition, to build and monitor non-local quantum coherence, and to implement methods for protecting non-local correlations against decoherence.
Results and achievements:
Quantum thermodynamics
Publications
♦ Alternative experimental ways to access entropy production
Z. Tan, P. A. Camati, G. Cœuret Cauquil, A. Auffèves, and I. Dotsenko
Phys. Rev. Research 3, 043076 (2021)
♦ Autonomous Maxwell’s demon in a cavity QED system
B.-L. Najera-Santos, P. A. Camati, V. Métillon, M. Brune, J.-M. Raimond, A. Auffèves, and I. Dotsenko
Phys. Rev. Research 2, 032025(R) (2020)
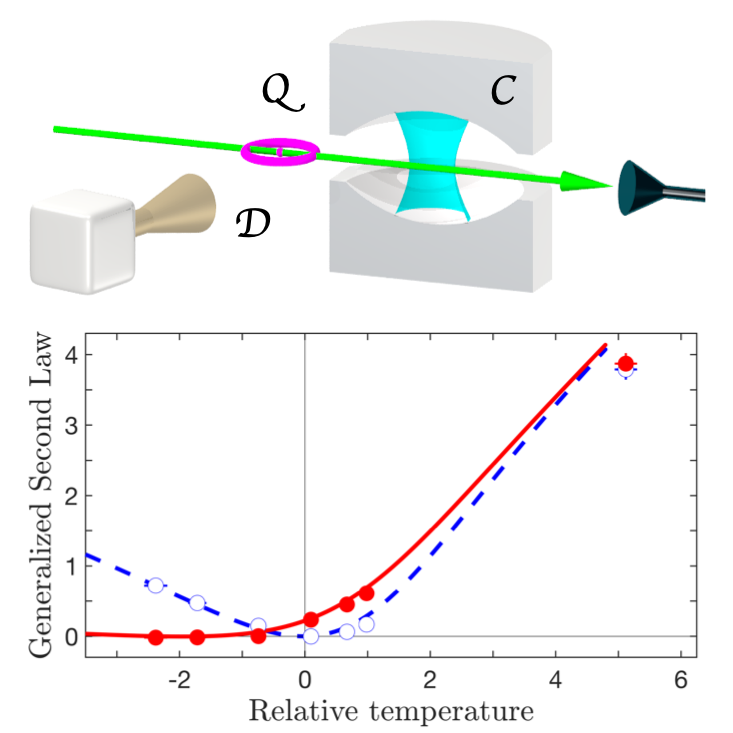
We report on the experimental realization of an autonomous Maxwell’s demon in a cavity QED system composed of a high-quality microwave resonator and circular Rydberg atoms. The modelled system is a qubit Q exchanging heat with a cavity C under the control of a demon D. Both Q and D are coded with a single three-level atom. The demon feedback is implemented by an auxiliary microwave source transferring the atomic population into the dark state, thus preventing the following QC interaction. The experimental ability to access independently the states of all system components allows us to fully characterize the energy, entropy and information flow in the system. While the classical expression for the Second Law seems to be violated, its generalized expression which includes the information acquired by D on the QD sub-system is respected.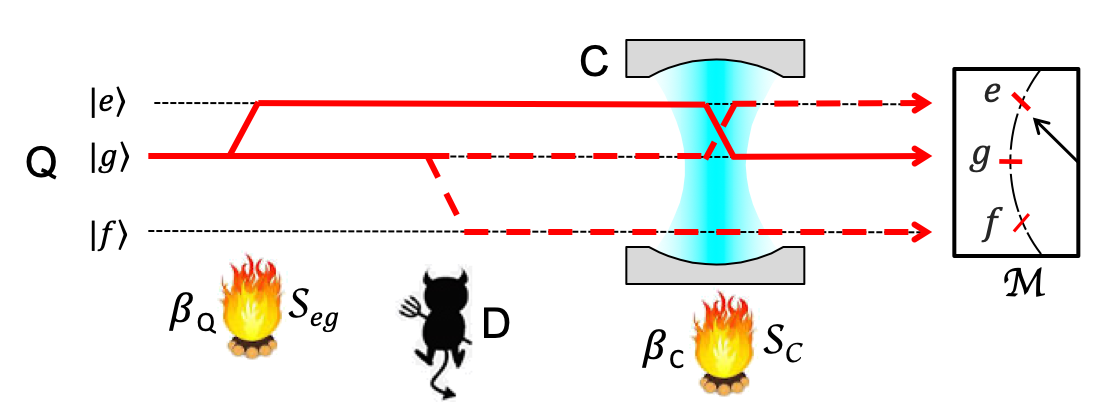
We propose also several different ways to access the entropy production in the QDC protocol. By recording and counting the evolution of individual trajectories, we independently measure EP with trajectory probabilities, heat and information transfer, relative entropy and mutual information between the system’s components, etc.
Quantum tomography of nonlocal states
Publications
♦ Benchmarking Maximum-Likelihood State Estimation with an Entangled Two-Cavity State
V. Métillon, S. Gerlich, M. Brune, J. M. Raimond, P. Rouchon, and I. Dotsenko
Phys. Rev. Lett. 123, 060404 (2019)
Supplementary materials
Theses
Valentin Metillon (2019)
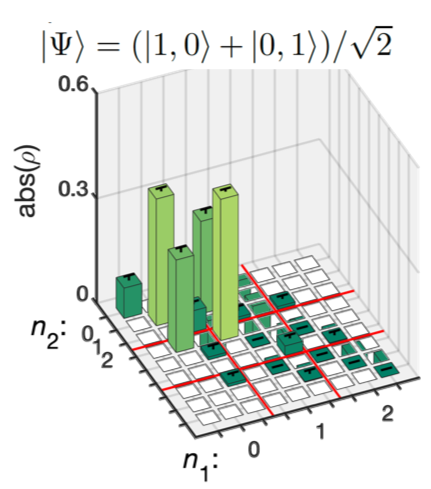
Quantum state estimation, or tomography, is a key component of quantum technologies, allowing to characterise quantum operations and to extract information on the results of quantum information processes. The usual tomography techniques rely on ideal, single-shot measurements of the unknown state. In this work, we use a new approach, called trajectory quantum tomography, where the quantum trajectory of each realization of the state is recorded through a series of measurements, including experimental imperfections and decoherence. This strategy increases the extracted amount of information and allows to build new measurements for a set of feasible measurements.
Using the tools of cavity quantum electrodynamics, we have prepared entangled states of microwave photons spread on two separated modes. We have then performed a trajectory tomography of these states, in a large Hilbert space. We have proved that this method allows to estimate the state, to develop faster strategies for extracting information on specific coherences of the state, and to compute error bars on the components of the estimated density matrix.
Quantum metrology beyond Standard Quantum Limit
Publications
♦ Measurement of a microwave field amplitude beyond the standard quantum limit
M. Penasa, S. Gerlich, T. Rybarczyk, V. Metillon, M. Brune, J.M. Raimond, S. Haroche, L. Davidovich, and I. Dotsenko
Phys. Rev. A 94, 022313 (2016)
arXiv:1605.09568 [quant-ph]
Supplementary materials
Theses
Mariane Penasa (2016)
As an essential intermediary between theories and their experimental proofs, measurement is meaningful if the precision of its results is high. The main emphasis of metrology in laboratories is therefore on increasing as much as possible the precision of the experimental evaluation of a parameter. Quantum noise that affects the measurement establishes a quantitative limit on the maximal precision that can be achieved with classical states: the standard quantum limit (SQL). Quantum metrology aims at using quantum features to beat this limit and to approach the physically ultimate limit called Heisenberg limit.
This thesis presents a measurement strategy for an electromagnetic field containing less than one photon, which is based on the use of atom-field correlations in a cavity quantum electrodynamics experiment. The idea is to measure the amplitude of the small field by probing the disturbance caused on an entangled mesoscopic state that is already stored in the superconducting cavity. We demonstrated that our measurement strategy is in principle optimal thanks to two tools: the Fisher information (that depends on the measurement process) and the quantum Fisher information (that does not), which define the precision tanks to Cramér-Rao like equations. The measurement signal subsequently largely exceeded the level of accuracy obtained with classical states and we got as closed to the Heisenberg limit as the experimental imperfections allowed us. The achieved experimental precision, 2.4 dB below the SQL, is well understood in terms of experimental imperfections. This method could be transposed to other systems, particularly to circuit QED, for the precise measurements of weak forces acting on oscillators.
Adaptive QND measurements and Past quantum states
Publications
♦ Adaptive Quantum Nondemolition Measurement of a Photon Number
B. Peaudecerf, T. Rybarczyk, S. Gerlich, S. Gleyzes, J. M. Raimond, S. Haroche, I. Dotsenko, and M. Brune
Phys. Rev. Lett. 112, 080401 (2014)
♦ Forward-Backward analysis of the photon-number evolution in a cavity
T. Rybarczyk, B. Peaudecerf, M. Penasa, S. Gerlich, B. Julsgaard, K. Moelmer, S. Gleyzes, M. Brune, J.M. Raimond, S. Haroche and I. Dotsenko
Phys. Rev. A 91, 062116 (2015)
Supplementary materials
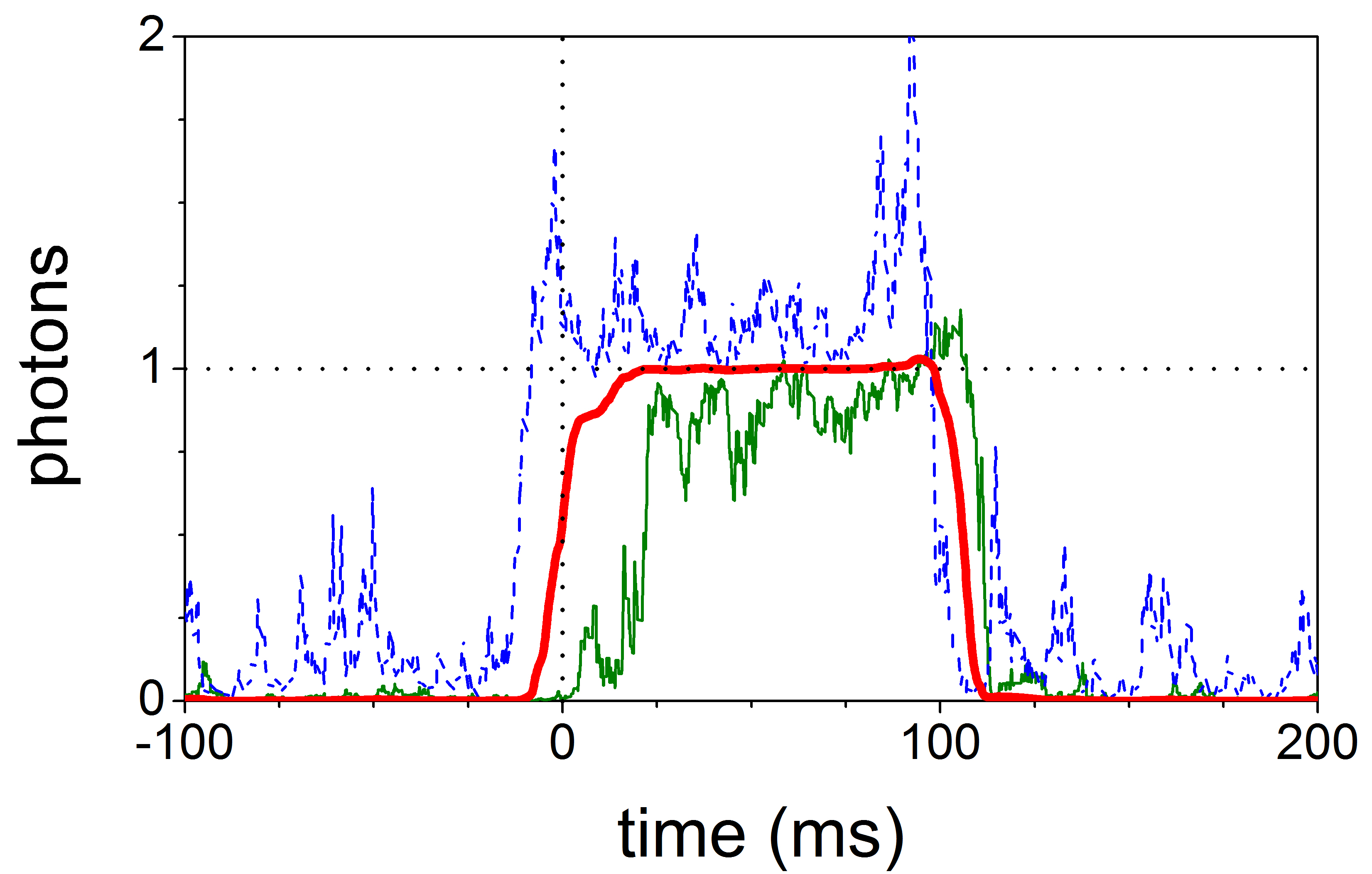
Using individual Rydberg atoms we can gain partial information on the field in the cavity. In order to extract the full information on the photon number and its evolution we have to use a long sequence of atoms interacting with the field one by one. In this way we have realized a basic QND photon number measurement. Recently we have significantly improved the quality of the extracted information by two upgrades: one to the measurement protocol realizing adaptive QND photon counting and the other to the data analysis applying past quantum state algorithm to the measured data.
In many quantum measurements, information is acquired incrementally by the successive interaction of meters with the measured system. Adaptive measurements minimize the use of resources (meters) by adjusting the measurement settings according to available information. We demonstrate an adaptive measurement for non-destructive photon counting in a cavity, based on Ramsey interferometry for Rydberg atoms interacting with the field. Tuning the interferometer in real time, we speed up the measurement by up to 45%. The criterion for the next measurement settings is the minimization of the expected state entropy after the measurement. This allows us not only to faster prepare quantum states by projection, but also to detect higher, and thus shorter-lived photon number states. Such adaptive methods are thus promising for quantum metrology, state preparation, and feedback.
Our quantum system is monitored through repeated interactions with atoms. The state of the system at time t, represented by the density matrix \(\rho(t)\), then becomes conditioned on the information obtained by the atoms until that time. More insight in the state at any time t is provided, however, by taking into account the full detection of all atoms interacting with the system both in the past and in the future of t. The application of the forward-backward smoothing method to our quantum problem, justified by the past quantum state formalism [S. Gammelmark et al., Phys. Rev. Lett. 111, 160401 (2013)], allows us to resolve a posteriori dynamics, which is not reflected by the usual quantum state \(\rho(t)\). This improved data analysis provides us very high precision of the cavity field evolution allowing us, for instance, to detect exact moments on quantum jumps and obtain more precise information on the photon number at any moment not influenced by quantum measurement noise. Besides, we have significantly removed the fundamental problem of any interferometric measurement, namely the ambiguity between very different states due to periodic nature of the measurement.
Quantum Feedback
Publications
♦ Real-time quantum feedback prepares and stabilizes photon number states
C. Sayrin, I. Dotsenko, X. Zhou, B. Peaudecerf, T. Rybarczyk, S. Gleyzes, P. Rouchon, M. Mirrahimi, H. Amini, M. Brune, J.-M. Raimond, and S. Haroche
Nature 477, 73-77 (2011)
♦ Field locked to a Fock state by quantum feedback with single photon corrections
X. Zhou, I. Dotsenko, B. Peaudecerf, T. Rybarczyk, C. Sayrin, S. Gleyzes, J. M. Raimond, M. Brune, and S. Haroche
Phys. Rev. Lett. 108, 243602 (2012)
♦ Quantum feedback experiments stabilizing Fock states of light in a cavity
B. Peaudecerf, C. Sayrin, X. Zhou, T. Rybarczyk, S. Gleyzes, I. Dotsenko, J. M. Raimond, M. Brune, and S. Haroche
Phys. Rev. A 87, 042320 (2013)
♦ Quantum feedback by discrete quantum nondemolition measurements : Towards on-demand generation of photon-number states
I. Dotsenko, M. Mirrahimi, M. Brune, S. Haroche, J.-M. Raimond, and P. Rouchon
Phys. Rev. A 80, 013805 (2009)
Supplemetary materials
The stability of complex systems, as well as many of our everyday actions rely on feedback loops. A probe measures the system state, a controller determines the action leading it towards the chosen operating point and an actuator realizes this action. Juggling, for instance, relies on numerous feedback loops from the eye to the hands through the brain.
Transferring the feedback concept to the quantum world is an important goal. Quantum physics allows one to realize tasks out of the reach of classical systems, such as precision measurements or complex information processing. The quantum states used for theses tasks are fragile, the most useful being also the most fragile. They must be protected. Feedback is a possible protection mechanism.
Quantum feedback must face a difficulty: quantum measurement changes the state of the system. The “Cavity Quantum Electrodynamics” group at LKB, in cooperation with Mines-ParisTech and INRIA, realized the first continuous quantum feedback experiment. They maintain a constant number of photons in a ’photon box’, a high-quality cavity. Atoms perform quantum measurements of the photon number, weak enough to avoid spoiling the state. The controller and the actuator correct the effect of this measurement and of the losses. Each loss of a photon in the environment is detected and corrected in real time. This experiment is an important step towards the control of complex and potentially useful quantum states.
♦ Quantum feedback trajectory stabilizing a 3-photon state. The movie shows the evolution of the density matrix estimated by the controller during one feedback trajectory lasting 164 ms (2000 loop iterations) as well as the evolution of the photon-number distribution for photon numbers from 0 to 7. The movie also features a sequence of the atom detection outcomes, estimated distance between the target and the actual state and, finally, applied corrections. Each movie frame corresponds to 2 feedback iterations.
A movie of decoherence

Publications
♦ Reconstruction of non-classical cavity field states with snapshots of their decoherence
S. Deléglise, I. Dotsenko, C. Sayrin, J. Bernu, M. Brune, J.-M. Raimond and S. Haroche
Nature 455, 510-514 (2008)
Supplementary materials
We are able to trap light in a « photon box » (a microwave cavity) for a time long enough to fully determine its quantum state and observe its evolution. This state is a mathematical object which allows physicists to predict the statistics of all possible measurements performed on the trapped photons. It can in general be represented by a map of values in a “phase plane” which looks as a three dimension geographical map. Each of its points, defined by its distance to the plane origin and its direction, is associated to a value of the light field amplitude and phase. Usual radiation (emitted by heated bodies, by lasers or by a combination of such sources) is quite generally described by a landscape of positive peaks centred at the points corresponding to the most probable values of the field. Physicists are now able to generate also non-classical fields, whose features are much less intuitive. Their maps present oscillations exhibiting negative values in some areas of the phase plane.
By probing the photon box with very sensitive atoms, the we have reconstructed the maps of these strange states. Fields with well defined photon numbers have maps presenting concentric oscillations. So called Schrödinger cat states, which are quantum superpositions of classical states with different phases are described by maps with two positive peaks corresponding to their classical components and, in between, a landscape of alternating positive ridges and negative valleys. By initially preparing these states in the box and recording their subsequent evolution,we have observed the progressive vanishing of the negative features. This reveals the fragility of the non-classical states which rapidly evolve into mundane classical states with positive maps.
Being able to reconstruct in details the dynamics of this decoherence phenomenon, which is at the heart of the quantum to classical boundary, opens the way to the manipulation and control of decoherence. Procedures of quantum-feedback become possible, in which atoms will be used to maintain in real time the non-classical features of a light field, and thus preserve the quantum properties which are essential for the realization of quantum information operations with light.
♦ Fifty milliseconds in the life of a Schrödinger cat. Movie of the reconstructed Wigner function of the even Schrödinger cat state. The state is reconstructed with the data recorded in a 4ms sliding time-window. The resulting Wigner functions are averaged over 4 ms. The movie exhibits two different phenomena: a fast decay of the quantum interference feature and a much slower evolution of the classical components towards phase-space origin. Fluctuations observed on top of the regular attenuation of the quantum interference term are due to statistical noise. Since successive frames are not independent, this noise has a 4 ms correlation time.
♦ Schrödinger cats’ quantumness vanishes. The even and odd cats have equal classical components and opposite quantum interferences. By subtracting their Wigner functions, we isolate the interference feature displaying their quantumness. Following the same procedure as in Video 1, we present the evolution of this signal over 50 ms which exhibits the fast decay, due to decoherence, of a pure interference pattern.
Freezing light: the quantum Zeno effect

Publications
♦ Freezing coherent field growth in a cavity by the quantum Zeno effect
J. Bernu, S. Deléglise, C. Sayrin, S. Kuhr, I. Dotsenko, M. Brune, J.-M. Raimond and S. Haroche
Phys. Rev. Lett. 101, 180402 (2008)
Supplementary materials
For the greek philosopher Zeno of Elea, motion was a paradoxical notion. At any time during its flight, an arrow is at some position, hence motionless. It thus cannot move at all! Nearly 2500 years later, physicists unveil a similar situation in quantum physics: the quantum Zeno effect. Repeated measurements on a quantum system can freeze its evolution. This effect is a direct consequence of the quantum measurement postulate, which states that each measurement projects with a high probability the system back on its initial state, freezing it efficiently. Observing this effect highlights the strangeness of the quantum measurement, which has puzzled us for nearly a century.
Quantum Zeno effect has been observed on the evolution of two-level systems. We have demonstrated it for the evolution of a small electromagnetic field, by repeatedly measuring the number of photons in a cavity through a Quantum Non Demolition process. The experiment, described in a Physical Review Letters paper, uses a microwave source which injects a coherent field in a very high quality superconducting cavity, initially empty. Without measurements, a coherent, classical field grows slowly in the cavity, following classical physics rules. When this growing field intensity is repeatedly measured, the situation is radically different. The measurement is realized with Rydberg atoms. They are so sensitive a probe of the microwave field that they can detect a microwave photon without absorbing it. The detection of each atom efficiently projects the field state back onto the initial vacuum state. The field evolution is thus freezed.
This paper has been published in Phys. Rev. Lett, with a ’News and views’ in Nature by A. Ourjoumtsev.
How to count and recount photons
Publications
♦ Progressive field-state collapse and quantum non-demolition photon counting
C. Guerlin, J. Bernu, S. Deléglise, C. Sayrin, S. Gleyzes, S. Kuhr, M. Brune, J.-M. Raimond and S. Haroche
Nature 448, 889 (2007)
♦ Process tomography of field damping and measurement of Fock state lifetimes by quantum nondemolition photon counting in a cavity
M. Brune, J. Bernu, C. Guerlin, S. Deléglise, C. Sayrin, S. Gleyzes, S. Kuhr, I. Dotsenko, J.-M. Raimond, and S. Haroche
Phys. Rev. Lett., 101, 240402 (2008)
♦ Optimal time-resolved photon number distribution reconstruction of a cavity field by maximum likelihood
C. Sayrin, I. Dotsenko, S. Gleyzes, M. Brune, JM. Raimond and S. Haroche
New J. Phys. 14, 115007 (2012)
Supplementary materials
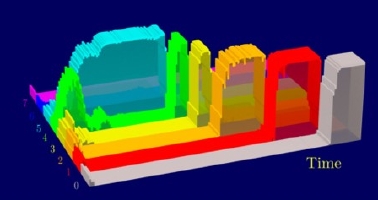
A quantum system can be in a superposition state in which a measurement result is not predetermined. An ideal quantum measurement cancels this quantum uncertainty. It leaves the system in a randomly chosen state (“God plays dice”), for which the measured quantity is precisely determined. We have recently realized such an ideal quantum measurement for the number of photons stored in a “box” (a microwave cavity made up of superconducting mirrors). The field is repeatedly probed by atoms, acting as microscopic clocks. The ticking of these clocks depend upon the photon number. Each atom thus carries partial information on the field. The accumulation of these partial measurements leads to the final collapse onto a quantum state in which the photon number (between 0 and 7) is perfectly determined. Correlations between repeated measurements assess the quality of this Quantum Non Demolition photon counting procedure. The quantum jumps corresponding to the escape of the photons, due to cavity damping, are directly observed. This experiment illustrates all quantum measurements postulates. It leads also to the preparation of interesting non-classical states.
Quantum jumps of light recording the birth and death of a photon in a cavity
Publications
♦ Quantum jumps of light recording the birth and death of a photon in a cavity
S. Gleyzes, S. Kuhr, C. Guerlin, J. Bernu, S. Deléglise, U. Busk Hoff, M. Brune, J.-M. Raimond and S. Haroche
Nature 446, 297 (2007)
Supplementary materials
Posters:
Quantum Non-Demolition measurement of light
Tools for fundamental cavity QED experiments
Theses:
Sébastien Gleyzes (2006)
A microscopic quantum system under continuous observation exhibits at random times sudden jumps between its states. The detection of this quantum feature requires a quantum non-demolition (QND) measurement repeated many times during the system’s evolution. Whereas quantum jumps of trapped massive particles (electrons, ions or molecules) have been observed, this has proved more challenging for light quanta. Standard photodetectors absorb light and are thus unable to detect the same photon twice. It is therefore necessary to use a transparent counter that can ’see’ photons without destroying them. Moreover, the light needs to be stored for durations much longer than the QND detection time. Here we report an experiment in which we fulfill these challenging conditions and observe quantum jumps in the photon number. Microwave photons are stored in a superconducting cavity for times up to half a second, and are repeatedly probed by a stream of non-absorbing atoms. An atom interferometer measures the atomic dipole phase shift induced by the non-resonant cavity field, so that the final atom state reveals directly the presence of a single photon in the cavity. Sequences of hundreds of atoms, highly correlated in the same state, are interrupted by sudden state switchings. These telegraphic signals record the birth, life and death of individual photons. Applying a similar QND procedure to mesoscopic fields with tens of photons should open new perspectives for the exploration of the quantum-to-classical boundary.
Those results have been published in Nature